Embark on an enlightening journey into the realm of geometry with our comprehensive Unit 1 Foundations of Geometry Answer Key. This meticulously crafted guide provides a solid foundation for understanding the fundamental concepts, theorems, and applications of geometry, empowering you to conquer geometric challenges with confidence.
Delve into the intricate world of points, lines, planes, and angles, unraveling the mysteries of geometric shapes and their properties. Master the art of geometric transformations, witnessing their profound effects on shapes. Explore the intricacies of measurement in geometry, deciphering the secrets of length, area, and volume.
Key Concepts in Unit 1 Foundations of Geometry
Geometry is the branch of mathematics that deals with the properties and relationships of shapes and figures in space. It is a fundamental subject that has applications in many fields, such as architecture, engineering, and art.
The basic concepts of geometry include points, lines, planes, and angles. Points are dimensionless and represent locations in space. Lines are one-dimensional and extend infinitely in both directions. Planes are two-dimensional and extend infinitely in all directions. Angles are formed by two intersecting lines and are measured in degrees.
Geometric Shapes and Their Properties, Unit 1 foundations of geometry answer key
Geometric shapes are figures that have specific properties. Some of the most common geometric shapes include triangles, squares, circles, and cubes. Triangles are three-sided polygons with three angles. Squares are four-sided polygons with four right angles. Circles are two-dimensional shapes with all points equidistant from a central point.
Cubes are three-dimensional shapes with six square faces.
Geometric Transformations
Geometric transformations are operations that can be performed on shapes. The most common geometric transformations include translations, rotations, reflections, and dilations. Translations move shapes from one location to another. Rotations turn shapes around a fixed point. Reflections flip shapes over a line.
Dilations enlarge or shrink shapes.
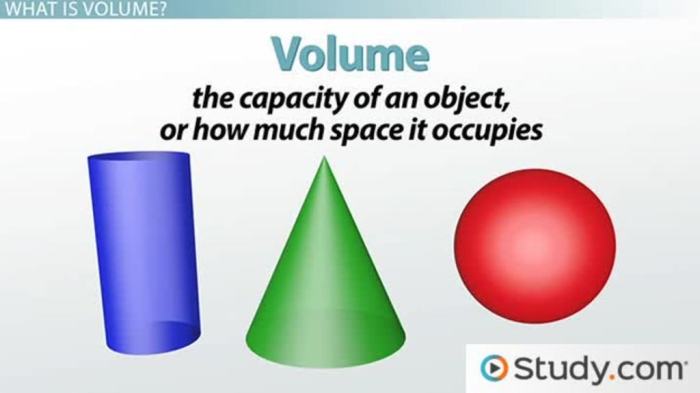
Measurement in Geometry
Measurement is the process of determining the size or quantity of something. In geometry, measurement is used to determine the length, area, and volume of shapes.
The most common units of measurement in geometry are the inch, the foot, the yard, and the mile. The inch is the smallest of these units, and the mile is the largest. Other units of measurement that are sometimes used in geometry include the centimeter, the meter, and the kilometer.
Formulas for Calculating Area and Volume
The area of a shape is the amount of space that it occupies. The volume of a shape is the amount of space that it encloses.
The following are formulas for calculating the area and volume of some common geometric shapes:
- Area of a triangle: A = ½ – base – height
- Area of a square: A = side²
- Area of a circle: A = π – radius²
- Volume of a cube: V = side³
- Volume of a sphere: V = 4/3 – π – radius³
Geometric Constructions

Geometric constructions are methods for creating geometric shapes using only a compass and a straightedge. The most common geometric constructions include bisecting an angle, constructing a perpendicular bisector, and constructing a parallel line.
Steps for Performing Geometric Constructions
The following are steps for performing some common geometric constructions:
- To bisect an angle, draw a ray that starts at the vertex of the angle and passes through the midpoint of the angle. The ray will divide the angle into two equal parts.
- To construct a perpendicular bisector, draw two circles that intersect at the midpoint of the line segment. The line segment that connects the two points of intersection will be perpendicular to the line segment.
- To construct a parallel line, draw a line that is parallel to the given line and passes through a point that is not on the given line. The two lines will be parallel.
Geometric Proofs: Unit 1 Foundations Of Geometry Answer Key
Geometric proofs are logical arguments that demonstrate the truth of geometric statements. Proofs are used to show that a statement is true for all possible cases.
There are two main types of geometric proofs: direct proofs and indirect proofs. Direct proofs prove a statement by showing that it is true for all possible cases. Indirect proofs prove a statement by showing that the opposite of the statement is false.
Examples of Geometric Proofs
The following is an example of a direct proof:
Theorem: The sum of the interior angles of a triangle is 180 degrees.
Proof: Let ABC be a triangle. Draw a line from vertex A to vertex C. This line will divide the triangle into two smaller triangles, ABD and ACD. The sum of the interior angles of triangle ABD is 180 degrees. The sum of the interior angles of triangle ACD is also 180 degrees. Therefore, the sum of the interior angles of triangle ABC is 180 degrees.
The following is an example of an indirect proof:
Theorem: There is no largest prime number.
Proof: Suppose that there is a largest prime number, p. Then consider the number p + 1. This number is either prime or composite. If p + 1 is prime, then it is a larger prime number than p, which contradicts our assumption that p is the largest prime number. If p + 1 is composite, then it has a prime factor, q. This prime factor must be less than p, since p is the largest prime number. But this contradicts the fact that q is a prime factor of p + 1. Therefore, our assumption that there is a largest prime number must be false.
FAQs
What is the significance of geometric constructions?
Geometric constructions play a crucial role in solving geometric problems and demonstrating theorems. They allow us to create precise geometric figures and explore their properties, facilitating a deeper understanding of geometric relationships.
How do I determine the similarity of two shapes?
To determine the similarity of two shapes, you can use the criteria for similarity, such as the Side-Side-Side (SSS) criterion, Side-Angle-Side (SAS) criterion, or Angle-Side-Angle (ASA) criterion. These criteria establish specific conditions under which two shapes are similar, ensuring proportional sides and congruent angles.
What is the relationship between congruence and similarity?
Congruence is a stronger relationship than similarity. Two congruent shapes are not only similar but also have equal dimensions. In other words, they are identical in size and shape, making them interchangeable without affecting the geometric properties of the figure.